階數

A polynomial is a function like (y= 3x3-2x+1). The degree of the polynomial is the largest power of the variable. For example, the degree of (3x3-2x+2) is 3; the degree of (-x5+x2) is 5, and so on. NURBS functions are rational polynomials and the degree of the NURBS is the degree of the polynomial. From a NURBS modeling point of view, the (degree –1) is the maximum number of "bends" you can get in each span.

例如：

A line has degree 1. It has zero bends.

1 階：直線。

拋物線、雙曲線、圓弧、圓 (圓錐斷面曲線) 為 2 階曲線，可以"彎曲" 1 次。

2 階：雙曲線、圓弧、圓。

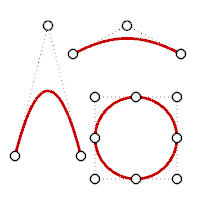
貝茲曲線屬於 3 階曲線，如果您將它的控制點排成 Z 形，曲線共彎曲了兩次。

3 階曲線。